Cylinder - fundamental group
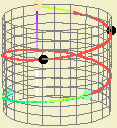
In the cylinder (that is, a cylindrical surface), the study of path homotopies is not as simple as in the plane. That becomes clear when we consider a closed path around the cylinder. As we cannot move the initial and end points of the path, it is not possible to contract the path to a point, then, these two paths, the closed path and the base point, are non-homotopic. This idea can be explored in the app.
Taking into consideration this example after exploring the app, what can we conjecture about homotopy classes of paths in the cylinder?
One important observation is that the homotopy classes depend on the number of times the paths representing them go around the surface. In fact, one can show that this determines each class. Given two paths, there is an homotopy between them if and only if the number of times each path goes around the cylinder is the same (if necessary, after completing the paths by a shortest segment connecting the initial and end poits of each).
Then, considering closed paths in a cylinder, an homotopy class is characterized by one integer. In this way, there is a correspondence between the homotopy classes and the integers - the fundamental group of the cylinder is isomorphic to \(\mathbb{Z}\).
...
...
This correspondence can also be explained by the lift of the cylinder to a planar strip. After choosing a base point in the cylinder and an initial point in the rectangle, each path in the cylinder admits a single lift with the chosen base point in the rectangle. Hence, the equivalence class of paths in the cylinder is determined by the end point of the corresponding path in the strip; this point can take countable infinitely many values, each of them determined by an integer (with respect to its position to the right or left of initial point).